Hello there. To solve this question, we'll have to remember some properties about vertical angles.
Given two angles A and B, they are said to be vertical angles if and only if:
That is, their measures are the same.
In this question, we have that the angles A and B have measures respectively equal to:
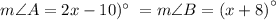
Solving this equation for x:
Subtract x on both sides of the equation. Add 10 in the same manner.
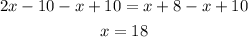
Therefore we have the solution for x.
Now we plug this into A in order to find its measure:

This is the answer to this question.