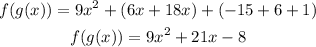Question

given definition of f (x) and g (x) below find the value of (f o g)f(x)=x squared - 6x - 15g(x)=-3x-1

Answer 1

The functions are

`f(x)=x^2-6x-15`
`g(x)=-3x-1`

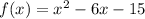
You have to calculate (f o g)(x), this means that you have to replace g(x) inside f(x) → f(g(x))

So for f(x) x will be g(x) as:

`\begin{gathered} f(g(x))=(g(x))^2-6(g(x))-15 \\ f(g(x))=(-3x-1)^2-6(-3x-1)-15 \end{gathered}`

I'll separate the composition in parts and solve them separatelly, once all terms are solved I'll add them together again:

So first solve the square of the binomial:

`\begin{gathered} (-3x-1)^2 \\ (-3x-1)(-3x-1) \\ (-3x)(-3x)+(-3x)(-1)+(-1)(-3x)+(-1)(-1) \\ 9x^2+3x+3x+1 \\ 9x^2+6x+1 \end{gathered}`

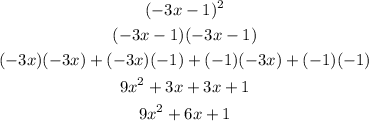
Next solve the second term, by applying the distributive property of multiplication:

`\begin{gathered} -6(-3x-1) \\ (-6)(-3x)+(-6)(-1) \\ 18x+6 \end{gathered}`

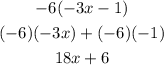
Now put both solutions toghether with the last term of the equation and order the like terms together:

`\begin{gathered} f(g(x))=(-3x-1)^2-6(-3x-1)-15 \\ f(g(x))=(9x^2+6x+1)+(18x+6)-15 \\ f(g(x))=9x^2+6x+18x-15+6+1 \end{gathered}`

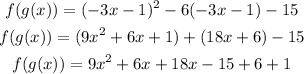
And finally simplify the expression by solving the operations between the like terms:

`\begin{gathered} f(g(x))=9x^2+(6x+18x)+(-15+6+1) \\ f(g(x))=9x^2+21x-8 \end{gathered}`