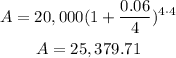Answer:

Explanation:
Compound interest is represented by the following expression:
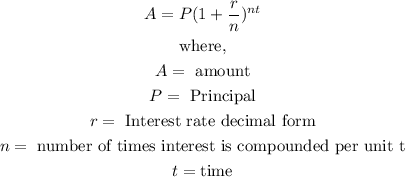
Then,
a) Compounded semiannually:
If semi-annually, then n=2.
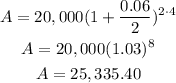
b) Compounded monthly:
If monthly, then n=12
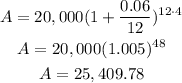
c) Compounded continuously:
If continuously (daily), then n=365
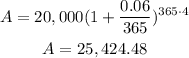
d) Compounded quarterly:
If quarterly, then n=4.