Question

PLEASE HELP ME Given that the 3rd term of a geometric sequence is 45 and the 6th term is the same sequence is 1215, write a formula for the general term.

Answer 1

Given:

`\begin{gathered} a_3=45 \\ a_6=1215 \end{gathered}`

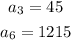
The formula for general term is:

`a_n=a_1r^(n-1)`

Thus, we can write:

`\begin{gathered} a_3=a_1r^2=45 \\ a_6=a_1r^5=1215 \end{gathered}`

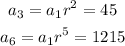
We can divide a_6 by a_3:

`\begin{gathered} (a_6)/(a_3) \\ (a_1r^5)/(a_1r^2)=(1215)/(45) \\ (r^5)/(r^2)=27 \\ r^(5-2)=27 \\ r^3=27 \\ r=\sqrt[3]{27} \\ r=3 \end{gathered}`

We found r = 3.

Now, we need first term, a_1.

So,

`\begin{gathered} a_3=a_1r^2=45 \\ a_1(3)^2=45 \\ 9a_1=45 \\ a_1=(45)/(9) \\ a_1=5 \end{gathered}`

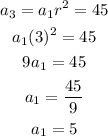
So,

First Term = 5

Common Ratio = 3

The general term of this geometric sequence is:

So,

First Term = 5

Common Ratio = 3

The general term of this geometric sequence is: