Question

Use the number line below, where RS = 4y + 3, ST = 3y + 9, and RT = 54.a. What is the value of y?b. Find RS and ST.

Answer 1

Let us make the line from the picture, pointing the given equations:

From the draw and the given equations, we note that:

`54=RT=RS+ST=4y+3+3y+9`

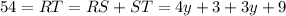
Therefore, we can solve the equation for y as follows

`\begin{gathered} 54=4y+3+3y+9 \\ \\ 54=7y+12 \end{gathered}`

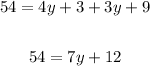
Where in the last line, we add the similar terms, for example 3y+4y=7y. Let us continue the calculations

`\begin{gathered} 54=7y+12 \\ \\ 54-12=7y \\ \\ 42=7y \end{gathered}`

where in the second line of the equation above, we pass the 12 from the right to the left with opposite sign. We discover y as follows

`\begin{gathered} 42=7y \\ \\ (42)/(7)=y \\ \\ 6=y \end{gathered}`

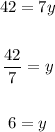
That is, we have y=6.

Let us now calculate the value of RD an RT. We know from the question that

`\begin{gathered} RS=4y+3 \\ \\ ST=3y+9 \end{gathered}`

as we discover that y=6, substituting this value in the equations above we find:

`\begin{gathered} RS=4*\text{ }6+3=24+3=27 \\ \\ ST=3*6+9=18+9=27 \end{gathered}`

We conclude that RS=27 and ST =27 .