Question

Ryan's age is 4 less than five times Joshua's age. The product of their ages is 105. Determine algebraically theages of Ryan and Joshua.

Answer 1

We will have the following:

From the problem we obtain the following expressions:

`\begin{gathered} R=5J-4 \\ \\ R\ast J=105 \end{gathered}`

Now, we will determine the ages, that is:

First, we replace the value of "R" in the second expression:

`\begin{gathered} (5J-4)(J)=105\Rightarrow5J^2-4J-105=0 \\ \\ \Rightarrow J=(-(-4)\pm√((-4)^2-4(5)(-105)))/(2(5)) \\ \\ \Rightarrow J=-4.2 \\ \\ and \\ \\ \Rightarrow J=5 \end{gathered}`

Now, since it would certainly make little sense to have a negative age we will take the positive value for Joshua's age, then:

`R=5(5)-4\Rightarrow R=21`

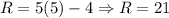
So, Ryan's age is 21 and Joshua's age is 5.

***Explanation***

We are told that Ryan's age is 4 less than 5 times Joshua's age. So, we can write Ryan's age in terms of Joshua's, that is:

`R=5J-4`

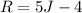
Here "R" is Ryan's age and "J" is Joshua's age.

We are also told that the product of their ages is 105. This can be expressed as:

`R\ast J=105`

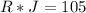
But we remember that Ryan's age can be written in terms of Joshua's age, and so, we obtain that:

`(5J-4)(J)=105`

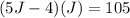
Now, we expand this expression, that is:

`\begin{gathered} (5J)(J)+(-4)(J)=105 \\ \\ \Rightarrow5J^2-4J=105 \end{gathered}`

From this, we can see that it can be written as the general quadratic form, that is:

`=5J^2-4J-105=0`

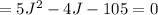
And thus, we can solve for "J" using the quadratic formula.

Note: Quadratic formula:

For a quadratic function that follows:

`ax^2+bx+c=0`

We can solve for the variable using the quadratic formula, that is:

`x=(-b\pm√(b^2-4ac))/(2a)`

And thus two solutions will be obtained:

`\begin{gathered} x=(-b-√(b^2-4ac))/(2a) \\ \\ and \\ \\ x=(-b+√(b^2-4ac))/(2a) \end{gathered}`

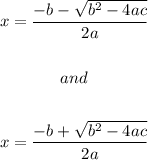
Now that we have recalled the quadratic formula, we will solve for the expression at hand:

`5J^2-4J-105=0`

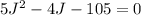
That is:

`J=(-(-4)\pm√((-4)^2-4(5)(-105)))/(2(5))`

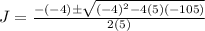
So, the two solutions will be:

`\begin{gathered} J=-4.2 \\ \\ and \\ \\ J=5 \end{gathered}`

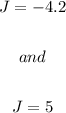
So, we have two values that can be used; but since a negative age makes no sense we only use the positive value; from this we can conclude that Joshua's age is 5 years.

Now that we know Joshua's age, we can replace it in Ryan's expression in terms of Joshua's age, that is:

`\begin{gathered} R=5J-4\Rightarrow R=5(5)-4 \\ \\ \Rightarrow R=25-4\Rightarrow R=21 \end{gathered}`

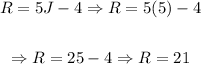
So, from this we can see that Ryan's age is 21 years.