ANSWER
Length: 23 ft
Width: 9 ft
Step-by-step explanation
The area of a rectangle is:
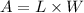
Where L and W are length and width. We know that for this rectangle the area is 207 ft²
and the length is 5 more than twice its width:
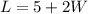
We have a system of equations:
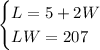
We can replace L from the first equation into the second equation:
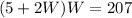
And solve for W. To do this we have to rewrite the equation above:
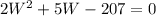
We have a quadratic equation, that can be solved with:
![\begin{gathered} ax^2+bx+c=0 \\ x=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/uuterq6bz1kwr2mb9jy58c523r5v4v644y.png)
In this case x = W, a = 2, b = 5 and c = -207:
![\begin{gathered} W=\frac{-5\pm\sqrt[]{5^2-4\cdot2\cdot(-207)}}{2\cdot2} \\ W=\frac{-5\pm\sqrt[]{25+1656}}{4} \\ W=\frac{-5\pm\sqrt[]{1681}}{4} \\ W=(-5\pm41)/(4) \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/pzpn9nqfcagk2fzwg479vecwna8b5cq93v.png)
Note that we have here two results, one negative and one positive. The only valid result is the positive one, because the width can't be negative:
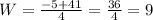
The width of the rectangle is 9 feet.
Then, to find the length we just have to replace the width into the first equation:
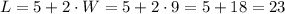
The length is 23 feet.