Answer:
The balance after the end of 10 years is $6,554.47
Step-by-step explanation:
The amount after t years is given as:

Principal, P = $4000
Interest rate, r = 5% = 5/100 = 0.05
Number of times compounded annually, n = 2
Time, t = 10 years
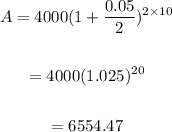
The balance after the end of 10 years is $6,554.47