Question

If an object fell 87 ft in 3 seconds, how far will it have fallen by the end of 6 seconds? Leave variation constant in fraction form or round two at least two decimal places. Round your final answer to the nearest foot.

Answer 1

we need to find the equation, so when they say is "directly proportional" means that when one variable grows, the other one will grow too. so here we have d (distance) and t^2 (square of the time) and proportional mean:

`d=at^2`

a is a constant we can calculate

we know when d=87 t=3

so we can replace and find the value of a

`\begin{gathered} 87=a\cdot3^2 \\ 87=a\cdot9 \\ (87)/(9)=a \end{gathered}`

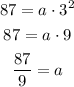
so the equation is

`d=(87)/(9)t^2`

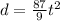
finally, we can replace t=6 and find the distance

`\begin{gathered} d=(87)/(9)\cdot6^2 \\ d=(87)/(9)\cdot36 \\ d=348 \end{gathered}`

so the answer is: 348 ft