Answer:
9x+15y=270
• (0,18): 0 short-sleeved shirts and 18 long-sleeved shirts.
,
• (30,0): 30 short-sleeved shirts and 0 long-sleeved shirts.
,
• (10,12): 10 short-sleeved shirts and 12 long-sleeved shirts.
Explanation:
Let the number of short-sleeved t-shirts sold = x
Let the number of long-sleeved t-shirts sold = y
• A Short-sleeved t-shirt costs $9, therefore x t-shirts costs $9x
,
• A long-sleeved t-shirt costs $15, therefore y t-shirts cost $15y
,
• At the game, she made $270.
An equation that represents the number of sales during the period is:
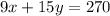
Next, the line is graphed.
First, find the intercepts.
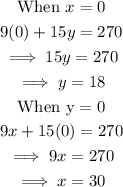
The intercepts are (0,18) and (30, 0).
The graph is attached below:
Three different combinations of shirts that could have been sold are:
0. (0,18): 0 short-sleeved shirts and 18 long-sleeved shirts.
,
1. (30,0): 30 short-sleeved shirts and 0 long-sleeved shirts.
,
2. (10,12): 10 short-sleeved shirts and 12 long-sleeved shirts.