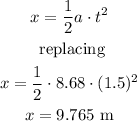Question

this is a 3 part question40) A 2.85-kg bucket is attached to a rope wrapped around a disk-shaped pulley of radius 0.121 m and mass 0.742 kg. If the bucket is allowed to fall, (a) what is its linear acceleration? (b) What is the angular acceleration of the pulley? (c) How far does the bucket drop in 1.50 s?

Answer 1

a) 8.68 m/s^2

b) 71.74 rad/s^2

c) 9.765 m

Explanation:

Given:

m1 = 2.85 kg

m2 = 0.742 kg

r = 0.121 m

a)

From the Newton's second law of motion, the downward force acting on the bucket is:

`\begin{gathered} \sum ^{}_{}F=m_1\cdot g-T=m_1\cdot a \\ T=m_1\cdot g-m_1\cdot a\text{ (1)} \end{gathered}`

Torque acting on the pulley is:

`\begin{gathered} \sum ^{}_{}\tau=I\alpha=rT \\ I\alpha=rT \end{gathered}`

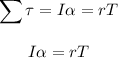
Hence, the angular acceleration is:

`\begin{gathered} \alpha=(a)/(r),\text{ and inertia is, I }=(1)/(2)m_2\cdot r^2 \\ \text{ therefore:} \\ (1)/(2)m_2\cdot r^2\cdot(a)/(r)=rT \\ T=(1)/(2)m_2\cdot a\text{ (2)} \end{gathered}`

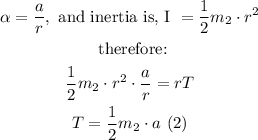
We equate equations (1) and (2), and solve for the acceleration like this:

`\begin{gathered} m_1\cdot g-m_1\cdot a=(1)/(2)m_2\cdot a \\ (1)/(2)m_2\cdot a+m_1\cdot a=m_1\cdot g \\ a\cdot((1)/(2)m_2+m_1)=m_1\cdot g \\ a=(m_1\cdot g)/((1)/(2)m_2+m_1) \\ \text{ replacing:} \\ a=(2.85\cdot9.81)/((1)/(2)\cdot0.742+2.85) \\ a=8.68m/s^2 \end{gathered}`

b)

The angular acceleration of the pulley is:

`\begin{gathered} \alpha=(a)/(r) \\ \text{ replacing} \\ \alpha=(8.68)/(0.121) \\ \alpha=71.74rad/s^2 \end{gathered}`

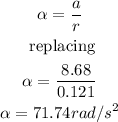
c)

We use the following equation to be able to calculate the distance:

`\begin{gathered} x=(1)/(2)a\cdot t^2 \\ \text{ replacing} \\ x=(1)/(2)\cdot8.68\cdot(1.5)^2 \\ x=9.765\text{ m} \end{gathered}`