Question

How many different committees can be formedfrom 6 teachersand 42 students if the committee consists of 3 teachers and 3 students?The committee of 6 members can be selected in different ways.

Answer 1

229,600ways

Given

The committee of six members consists of 3 teachers and 3 students

Solution

We need to choose 3 of the 6 teachers AND 3 of the 42 students:

`^nC_r=(n!)/((n-r)!* r!)`

where the combination is a selection of r possible combinations of objects from a set of n objects.

`\begin{gathered} (^6C_3)(^(42)C_3)=(6!)/((6-3)!*3!)*(42!)/((42-3)!*3!) \\ \\ (6!)/((6-3)!*3!)*(42!)/((42-3)!*3!)=\frac{6!^{}^{}^{}}{3!*3!}*(42!)/(39!*3!) \\ \\ \frac{6!^{}^{}^{}}{3!*3!}*(42!)/(39!*3!)=20*11480 \\ \\ \\ =229600 \end{gathered}`

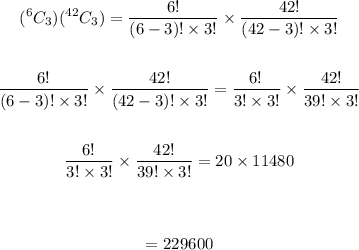
The Final Answer

The committee of 6 members can be selected in 229,600 different ways.