Question

The half-life of radio-active cesium-137 is 30 years. A quantity of this substance weighs 22 grams today. How much did it weigh 11 years ago?a. 20.96 gramsb. 11.27 gramsc. 28.37 gramsd. 27.06 gramse. 16.07 grams

Answer 1

The half-life of a compound is the time needed to the mass to reduce to half of its initial value. If x is the time in years, x₀ is the half-life or the compound, m₀ the initial mass, m the mass after x years, we can use the following formula:

`m=\frac{m_0}{2^{(x)/(x_0)}}`

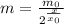
For m = 22 grams, x₀ = 30 years, and x = 11 years, we are looking for the value of m₀. From the first relation, we can isolate m₀ and substitute the values:

`\begin{gathered} m_0=m*2^{(x)/(x_0)} \\ m_0=22*2^{(11)/(30)}=22*2^(1.289)\cong28.37grams \end{gathered}`

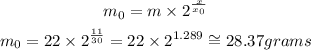
From the present solution, we conclude the answer is c. 28.37 grams.