a)
Angles FGH and HGI are equal [since GH bisects Angle FGI].
We can equate their algebraic expressions and solve for x first. Shown below:
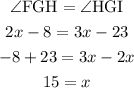
b)
We want to find Angle FGH, 2x - 8, found x = 15.
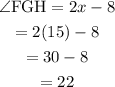
c)
We want to find HGI. Similar process as b). Shown below:

d)
Angle FGI is the sum of Angle FGH and Angle HGI, which are both found to be 22 each.
Thus,
