Question

Find the X- and Y- intercepts of the line that passes through the given points (-4, -4), (8, -1)the x intercept isthe y intercept is

Answer 1

To determine the y- and x- intercepts of the line that passes through the points (-4,-4) and (8,-1) you have to determine the equation of the line first. To do so, you have to use the point-slope form:

`y-y_1=m(x-x_1)`

Where

m represents the slope of the line

(x₁,y₁) represent the coordinates of one of the points of the line

The first step is to calculate the slope of the line, using the formula:

`m=(y_1-y_2)/(x_1-x_2)`

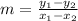
Where

(x₁,y₁) represent the coordinates of one of the points of the line

(x₂,y₂) represent the coordinates of a second point of the line

Using

(8,-1) as (x₁,y₁)

(-4,-4) as (x₂,y₂)

You can calculate the slope as follows:

`\begin{gathered} m=(-1-(-4))/(8-(-4)) \\ m=(-1+4)/(8+4) \\ m=(3)/(12) \\ m=(1)/(4) \end{gathered}`

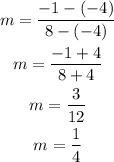
The slope of the line is m=1/4

Next, using the slope and one of the points, for example, point (8,-1) you can determine the equation of the line as follows:

`\begin{gathered} y-y_1=m(x-x_1) \\ y-(-1)=(1)/(4)(x-8) \\ y+1=(1)/(4)(x-8) \end{gathered}`

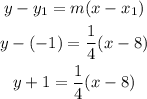
-distribute the multiplication on the parentheses term

`\begin{gathered} y+1=(1)/(4)\cdot x+(1)/(4)\cdot(-8) \\ y+1=(1)/(4)x-2 \end{gathered}`

-pass +1 to the right side of the equation by applying the opposite operation to both sides of it

`\begin{gathered} y+1-1=(1)/(4)x-2-1 \\ y=(1)/(4)x-3 \end{gathered}`

The y-intercept is the point where the line crosses the y-axis, at this point the value of x is zero. So replace the equation of the line with x=0 and calculate the corresponding value of y:

`\begin{gathered} y=(1)/(4)x-3 \\ y=(1)/(4)\cdot0-3 \\ y=-3 \end{gathered}`

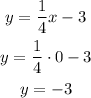
The coordinates of the y-intercept are (0,-3)

The x-intercept is the point where the line crosses the x-axis. At this point, the y-coordinate is zero. To determine the coordinates of the x-intercept you have to replace the equation of the line with y=0 and calculate the corresponding value of x:

`\begin{gathered} y=(1)/(4)x-3 \\ 0=(1)/(4)x-3 \end{gathered}`

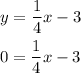
-Pass "-3" to the left side of the equation by applying the opposite operation to both sides of it:

`\begin{gathered} 0+3=(1)/(4)x-3+3 \\ 3=(1)/(4)x \end{gathered}`

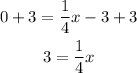
-Multiply both sides by 4 to determine the value of x

`\begin{gathered} 3\cdot4=4\cdot(1)/(4)x \\ 12=x \end{gathered}`

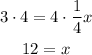
The coordinates of the x-intercept are (12,0)