Question

Directions: Given the following information, find and classily any relative extrema of / (x). Justify your answers.Question #6

Answer 1

Step 1:

Write the function

`f(x)=-x^3+6x^2-9x\text{ + 7}`

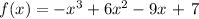
Step 2

To find the extremer, first, find the first derivative and then equate the first derivative to zero

`f^(\prime)(x)=-3x^2+12x\text{ - 9}`

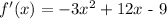
Step 3:

Equate the first derivative to zero to find the critical points

`\begin{gathered} -3x^2\text{ + 12x - 9 = 0} \\ x^2\text{ - 4x + 3 = 0} \\ x^2\text{ - x - 3x + 3 = 0} \\ x(x\text{ - 1) -3( x - 1) = 0} \\ (x\text{ - 3)(x - 1) = 0} \\ \text{x = 3 or 1} \end{gathered}`

Step 4

Next, find the extreme values

Finding the Absolute Extrema

Find all critical numbers of f within the interval [a, b]. ...

Plug in each critical number from step 1 into the function f(x).

Plug in the endpoints, a and b, into the function f(x).

The largest value is the absolute maximum, and the smallest value is the absolute minimum.

`\begin{gathered} f(x)=-x^3+6x^2-9x+7 \\ f(1)=-1^3\text{ + 6 }*1^2\text{ - 9}*1\text{ + 7= -1 + 6 - 9 +7 = }3 \\ f(3)\text{ = }-3^3\text{ + 6 }*3^2\text{ -9}*3\text{ + 7} \\ f(3)\text{ = -27 + 54 - 27 + 7 = 7} \end{gathered}`

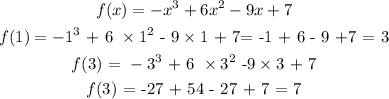
minimum (1 , 3) and maximum ( 3 , 7 )