A Right triangle is a triangle that has an angle whose measure is 90 degrees.
The Pythagorean Theorem states that:
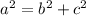
Where "a" is the hypotenuse (the longest side of the Right triangle) and "b" and "c" are the legs of the triangle.
Knowing the above, you can check each set:
Set A
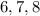
Notice that the longest side is 8. Then:
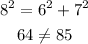
This set couldn't form a Right triangle.
Set B
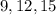
The longest side is 15. Then:
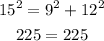
This set could form a Right triangle.
Set C
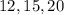
Knowing that the longest side is 20, you get:
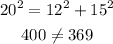
This set couldn't form a Right triangle.
Set D
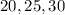
The longest side is 30. Then:
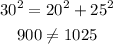
This set couldn't form a Right triangle.
The answer is: Option B.