SOLUTION:
Case: Trigonometry (Quadrants)
Given:

Required:
Find sin (2A)
Method:
Step 1: First we find the acute angle A, that sinA = 4/5
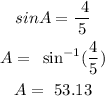
From here,
CosA is:
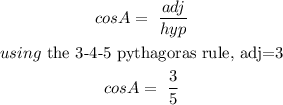
Step 2: Rotate the angle into the 3rd quadrant
A*= 53.13 + 180
A*= 233.13.
Step 3: Sin (2A)
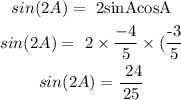
Final answer:
The value of sin(2A)= 24/25