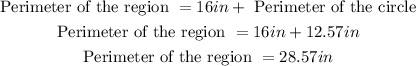The perimeter is the sum of all the sides of a geometric figure. To find the perimeter of this figure you can add the straight sides and then add the perimeter of a circle with a radius equal to 2.
Then, you have
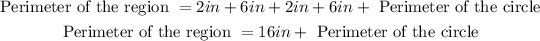
The formula to find the perimeter of a circle is
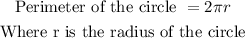
So,

Finally, the perimeter of the region will be 28.57 inches.