Question

For the piecewise function, find the values h( - 6), h(0), h(5), and h(9).- 5x – 13, for x < -3h(x) = { 5, for - 35x<5for x>5x+1,h(-6)=

Answer 1

Given the piecewise function h(x):

`h(x)=\begin{cases}-5x-13,x<-3 \\ 5,-3\leq x<5 \\ x+1,x\ge5\end{cases}`

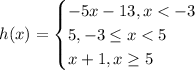
-When x is less than "-3", h(x)=-5x-13

-When x is between -3 and 5, h(x)=5

-When x is greater than or equal to 5, h(x)=x+1

1) For h(-6), this notation indicates that you have to determine the value of h(x) when x=-6

-6 is less than -3, which means that for this value of x, the function has the following shape

`h(x)=-5x-13`

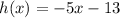
Replace the expression with x=-6 and calculate the corresponding value of x:

`\begin{gathered} h(-6)=-5(-6)-13 \\ h(-6)=30-13 \\ h(-6)=17 \end{gathered}`

2) For h(0), you have to determine the value of h(x) when x=0. Zero is between -3 and 5, for this value of x, the function h(x) has the following shape:

`h(x)=5`

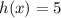
This equation represents a horizontal line, which means that for every value within the interval of definition -3≤x<5, the function always has the same value h(x)=5

We can conclude that:

`h(0)=5`

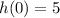
3) For h(5), you have to determine the value of h(x) for x=5, for values of x greater than or equal to 5, h(x) has the following shape:

`h(x)=x+1`

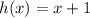
Replace the expression with x=5 and calculate the corresponding value of h(x):

`\begin{gathered} h(5)=5+1 \\ h(5)=6 \end{gathered}`

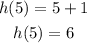
4) For h(9), you have to determine the value of h(x) when x=9, 9 is greater than 5, for this value of x, the function has the following shape:

`h(x)=x+1`

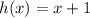
Replace the expression with x=9, and calculate the corresponding value of h(x):

`\begin{gathered} h(9)=9+1 \\ h(9)=10 \end{gathered}`

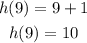
So, to sum up: