Question

A delivery truck is transporting boxes in 2 sizes: large and small. The large boxes weigh 45 pounds each and the small ones weigh 15 pounds each, There are 130 boxes in all. How many of each type of box is it carrying?

Answer 1

From the text, we can set a system of equations. Let's call the amount of large boxes "x" and the amount of small boxes "y".

From the sentence "There are 130 boxes in all." we get the following equation:

`x+y=130`

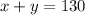
From the sentence "The truck is carrying a total of 4050 pounds in boxes" we get the following equation:

`45x+15y=4050`

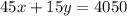
With this, we have our system.

`\begin{cases}x+y=130 \\ 45x+15y=4050\end{cases}`

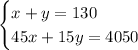
To make it easier to solve, we can divide the second equation by 15 and get the following system:

`\begin{cases}x+y=130 \\ 3x+y=270\end{cases}`

Subtracting the first equation from the second equation, we get a relation that depends only on the y value.

`\begin{gathered} 3x+y-(x+y)=270-(130) \\ 2x=140\Rightarrow x=70 \end{gathered}`

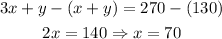
Now, if we just substitute our x value in any equation of the system, we get our y value.

`\begin{gathered} (70)+y=130 \\ \Rightarrow y=60 \end{gathered}`

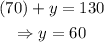
This means we have 70 large boxes and 60 small boxes.