Given data:
* The initial velocity of the ball is 25 m/s.
* The angle of the initial velocity with the horizontal is 60 degree.
* The final vertical velocity of the ball at the maximum height is 0 m/s.
Solution:
By the kinematics equation, the time taken by the projectile to reach the maximum height is,
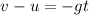
where v is the final vertical velocity at the maximum, u is the initial vertical velocity, g is the acceleration due to gravity, and t is the time taken to reach the maximum height,
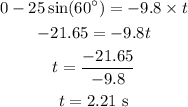
Thus, the time of flight of the ball is,
![undefined]()