Question

A cylinderical barrel is to hold 210L of industrial chemicals. Determine the radius and height of the barrel with the minimum surface areato the nearest tenth of a centimeter. (Recall: 1L=100cm3)

Answer 1

Let r be the radius (in cm) of the barrel, and h be its height (in cm), then we can set the following equations:

`\begin{gathered} \pi r^2h=21000\text{.} \\ SA=2\pi rh+2\pi r^2\text{.} \end{gathered}`

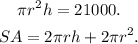
Solving the first equation for h we get:

`h=(21000)/(\pi r^2)\text{.}`

Substituting the above equation in the second equation we get:

`\begin{gathered} SA=2\pi r(21000)/(\pi r^2)+2\pi r^2, \\ SA=\frac{42000}{r^{}}+2\pi r^2\text{.} \end{gathered}`

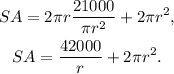
Now, we will use the first and second derivative criteria to obtain the minimum value for the above equation for the Surface Area.

The first and second derivatives of SA are:

`\begin{gathered} SA(r)^(\prime)=-(42000)/(r^2)+4\pi r, \\ SA(r)^(\doubleprime)=(84000)/(r^3)+4\pi\text{.} \end{gathered}`

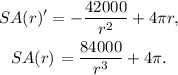
Setting SA(r)'=0 and solving for r we get:

`\begin{gathered} 0=-(42000)/(r^2)+4\pi r, \\ (42000)/(r^2)=4\pi r, \\ 42000=4\pi r^3, \\ r^3=(10500)/(\pi), \\ r\approx14.95. \end{gathered}`

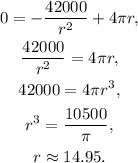
Evaluating SA''(r) at r=14.95 we get:

`SA^(\doubleprime)(14.95)_{}=(84000)/(14.95^3)+4\pi>0.`

Therefore, SA(r) reaches a local minimum at r≈15.0.

Substituting r=15.0 in h(r) we get:

`\begin{gathered} h(15.0)=(21000)/(\pi\cdot15.0^2), \\ SA(15.0)\approx29.7. \end{gathered}`

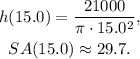
Answer: The radius is 15.0 cm and the height is 29.7 cm.