Question

How do I calculate the coefficient of friction of an object on an incline before the object starts moving? I have measured the angle at 45 degrees and the mass of the object on the incline is 250 g.

Answer 1

Given data:

* The angle of the inclined plane is

`\theta=45^(\circ)`

* The mass of the object is m = 250 g.

Solution:

The diagrammatic representation of the given case is,

The force acting down the inclined plane due to the weight of an object is,

`F=mg\cos (90^(\circ)-\theta)`

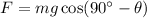
where g is the acceleration due to gravity,

Substituting the known values,

`\begin{gathered} F=250*10^(-3)*9.8*\cos (90^(\circ)-45^(\circ)) \\ F=0.25*9.8*\cos (45^(\circ)) \\ F=1.73\text{ N} \end{gathered}`

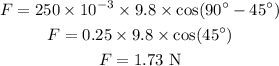
The normal force acting on the object is,

`\begin{gathered} F_N=mg\cos (\theta) \\ F_N=250*10^(-3)*9.8*\cos (45^(\circ)) \\ F_N=0.25*9.8*\cos (45^(\circ)) \\ F_N=1.73\text{ N} \end{gathered}`

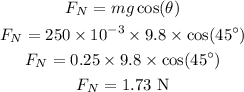
The frictional force in terms of the normal force is,

`F_s=\mu_sF_N`

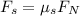
where,

`\mu_s\text{ is the static coefficient of friction}`

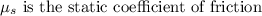
As the object is in a static state under the action of force, thus, the frictional force is balancing the force acting on the object down the inclined plane (F_s = F)

Substituting the known values,

`\begin{gathered} 1.73=\mu_s*1.73 \\ \mu_s=(1.73)/(1.73) \\ \mu_s=1 \end{gathered}`

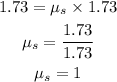
Hence, the coefficient of friction of an object on an inclined plane is 1.