Question

Let f(x)=x²−6x+13 . What is the vertex form of f(x)? What is the minimum value of f(x)? Enter your answers in the boxes. Vertex form: f(x)= Minimum value of f(x):

Answer 1

We need to write the vertex form of the quadratic function:

`f\mleft(x\mright)=x²−6x+13`

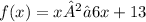
The vertex form of a quadratic function is written as:

`f(x)=a(x-h)^2+k`

where a is the leading coefficient and (h,k) is the vertex of the parabola.

We have:

`\begin{gathered} f(x)=x²−6x+13 \\ \\ f(x)=x²-2(3)x+13+9-9 \\ \\ f(x)=\lbrack x²-2(3)x+9\rbrack+13-9 \\ \\ f(x)=\lbrack x²-2(3)x+3²\rbrack+4 \\ \\ f(x)=(x-3)²+4 \end{gathered}`

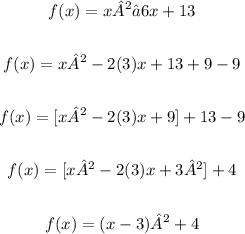
Thus, we obtained:

• a = 1

,

• h = 3

,

• k = 4

Since the leading coefficient is positive, the parabola opens upwards. Therefore, the y-coordinate of its vertex (k) is the minimum value of f(x).

Answer

Vertex form: f(x) = (x - 3)² + 4

Minimum value of f(x): 4