Question

Solve by substitution or elimination.10a+6b=8, 5a+3b=2

Answer 1

Given

`\begin{gathered} 10a+6b=8 \\ 5a+3b=2 \end{gathered}`

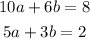
We are to Solve by substitution or elimination.

Solution

Using Elimination method

`\begin{gathered} 10a+6b=8\ldots Equation\text{ (i)} \\ 5a+\text{ 3b=2 }\ldots Equation(ii) \end{gathered}`

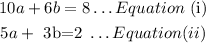
Multiply the second equation by -2, then add the equations together.

`\begin{gathered} 10a+6b=8 \\ -2(5a+3b=2) \\ \text{becomes} \\ 10a+6b=8 \\ -10a\text{ -6b=-4} \end{gathered}`

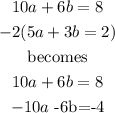
Add these equations to eliminate a:

`0=4`

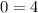
So the answer

No solutions.

Why it is no solution

A system has no solutions if two equations are parallel. ... When two equations have the same slope but different y-axis, they are parallel. Since there are no intersection points, the system has no solutions.