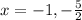Question

Solve for x. Write both solutions, separated by acomma.For the few questions in this set, you willsee the quadratic formula. Soon, you willneed to answer the questions withoutthis reminder.2x2 + 7x + 5 = 0Enter the correct answer.X=--+ b2 - 4ac2aO00DONEClear allDOOfor ax? + bx+c=0

Answer 1

Identify the values of a, b, and c, by comparing the given equation to the following.

`ax^2+bx+c=0`

Thus, the value of a, b, and c are as follows.

`\begin{gathered} a=2 \\ b=7 \\ c=5 \end{gathered}`

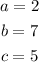
Substitute the values into the quadratic formula.

`\begin{gathered} x=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a} \\ x=\frac{-7\pm\sqrt[]{7^2-4(2)(5)}}{2(2)} \end{gathered}`

Simplify the exponential expression.

`x=\frac{-7\pm\sqrt[]{49^{}-4(2)(5)}}{2(2)}`

Multiply.

`x=\frac{-7\pm\sqrt[]{49^{}-40}}{4}`

Subtract.

`x=\frac{-7\pm\sqrt[]{9}}{4}`

Evaluate the radical expression.

`x=(-7\pm3)/(4)`

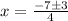
Rewrite the equation in two separate equations.

`\begin{gathered} x=(-7+3)/(4) \\ \\ x=(-7-3)/(4) \end{gathered}`

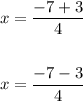
Simplify the numerators.

`\begin{gathered} x=(-7+3)/(4)=(-4)/(4)=-1 \\ \\ x=(-7-3)/(4)=-(10)/(4)=-(5)/(2) \end{gathered}`

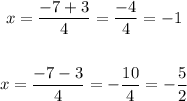
Thus,

`x=-1,-(5)/(2)`