Question

Solve. Show your work.log3 (2x + 1) = log3 (6x – 23)

Answer 1

`x=6`

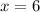
Step-by-step explanation:

To solve this, we need to use two properties of logarithms.

The first one is the difference of logarithms with same base:

`\log_a(x)-\log_a(y)=\log_a((x)/(y))`

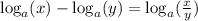
The second one is the definition of logarithm:

`\log_aN=x\Leftrightarrow N=a^x`

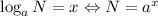
Then, we are given the equation:

`\log_3(2x+1)=\log_3(6x-23)`

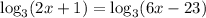
Then:

Applying the first property, for difference of logs:

Now we apply the definition of log:

`\log_3((2x+1)/(6x-23))=0\Leftrightarrow(2x+1)/(6x-23)=3^0`

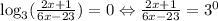
And now we can solve as any rational equation:

`\begin{gathered} (2x+1)/(6x-23)=3^0 \\ . \\ (2x+1)/(6x-23)=1 \\ . \\ 2x+1=1(6x-23) \\ 2x+1=6x-23 \\ 1+23=6x-2x \\ 24=4x \\ . \\ x=(24)/(4)=6 \end{gathered}`

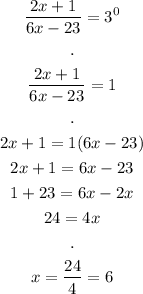
Thus, the answer is x = 6