8000 Newtons
Step 1
find the acceleration
the acceleration can be calculated by using the formula:
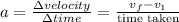
then,let
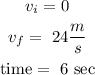
now, replace in the formula

Step 2
find the force.
Newton's Second Law of Motion says that acceleration happens when a force acts on a mass (object), it is given by:
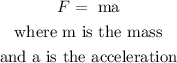
then, let
![\begin{gathered} m=2.0\cdot10^3\operatorname{kg} \\ a=4\text{ }(m)/(s^2) \end{gathered}]()
now, replace
![\begin{gathered} F=ma \\ F=2.0\cdot10^3\operatorname{kg}\cdot4(m)/(s^2) \\ F=8000\text{ Newtons} \end{gathered}]()
therefore , the answer is
8000 Newtons
I hope this helps you