Answer:
26 years
Step-by-step explanation:
The amount at the end of t years can be calculated as:
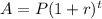
Where P is the principal and r is the interest rate.
If we want to find the doubling time, we need to replace A by 2P and solve for t, so we get:
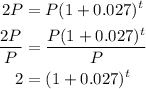
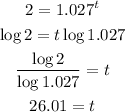
Therefore, the doubling time for this situation is approximately 26 years.