Given
Population of the world in 1987 = 5 billion
Annual growth rate = 1.3% per year
Required: The projected population in 2020
The exponential population growth formula is defined as:
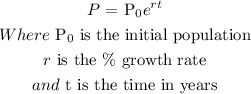
Substituting the given values:

After 2020, t = 33 years
Hence, the population after 33 years is:

Hence, the estimated population of the world in 2020 is 7678605172.0