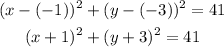Question

Find the equation for the circle with center ( - 1. - 3) and passing through (-5,2).Which is the correct equation?A. (x + 1)2 + (y + 3)2 = 36B. (x - 1)2 + (y - 3)2 = 25C. (x - 113 + (y - 3)2 = 13OD. (x + 1)2 + (y + 3)2 = 41

Answer 1

The equation of the circle with center at (h, k) and radius r is:

`(x-h)^2+(y-k)^2=r^2`

In this case, the center (-1, -3) means that h = -1, and k = -3. Given that the circle passes through (-5, 2), then the values x = -5, and y = 2 satisfies the equation. Substituting these values into the general equation, we get:

`\begin{gathered} (-5-(-1))^2+(2-(-3))^2=r^2 \\ (-5+1)^2+(2+3)^2=r^2 \\ (-4)^2+(5)^2=r^2 \\ 16+25=r^2 \\ 41=r^2 \end{gathered}`

Finally, the equation of the circle is:

`\begin{gathered} (x-(-1))^2+(y-(-3))^2=41 \\ (x+1)^2+(y+3)^2=41 \end{gathered}`