Question

A. A portable heater, rated at 1 kW, is plugged into a 120 V outlet. How many amperes will it draw? B. What amount of charge will be delivered in 1.0 minutes? C. How many electrons will be transferred? D. Find the energy delivered to the heater? E. What is the resistance of the heater? F. If the current exceeds 10 A, the heater will burn out. If it is plugged into a 220 V circuit, will it burn out?

Answer 1

Part A)

The power (P) delivered by an electric current (I) passing through a voltage (V) is given by the equation:

`P=VI`

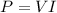
The power P and the voltage V are given, and the current I is unknown. Isolate I from the equation and replace P=1kW and V=120V to find the magnitude of the current that the portable heater will draw:

`I=(P)/(V)=(1kW)/(120V)=(1000W)/(120V)=8.33A`

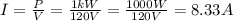
Therefore, the portable heater will draw 8.33 Amperes.

Part B)

The electric current (I) is the rate of transfer of electric charge (Q) per unit time (t):

`I=(\Delta Q)/(\Delta t)`

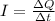
On the other hand, a current of one Ampere transfers a Coulomb of charge in one second. Isolate ΔQ from the equation, replace I=8.33A and Δt=1.0min=60s to find the charge delivered in 1.0 minute:

`\Delta Q=I\cdot\Delta t=(8.33A)(60s)=500C`

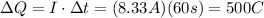
Therefore, 500 Coulomb of charge will be delivered to the portable heater in 1.0 minute.

Part C)

Let N be the number of electrons transferred to the heater. If e is the charge of a single electron, and a total charge of 500 Coulomb is transferred to the heater, then:

`e* N=500C`

Isolate N from the equation:

`N=(500C)/(e)`

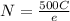
Replace the value of the charge of a single electron to find the number of electrons:

`e=1.602*10^(-19)C`

Then:

`N=(500C)/(1.602*10^(-19)C)=3.12*10^(21)`

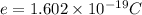
Then, 3.12*10^21 electrons are transferred.

Part D)

Since power is the rate of transfer of energy with respect to time, then:

`P=(\Delta E)/(\Delta t)`

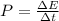
Isolate ΔE and replace P = 1kW and Δt = 60s to find the total energy delivered to the heater:

`\Delta E=P\cdot\Delta t=(1kW)(60s)=(1000W)(60s)=60,000J`

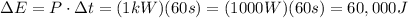
Therefore, the energy delivered to the heater is 60,000 Joules.

Part E)

The resistance of a circuit is defined as the quotient between the voltage of the circuit and the current that passes through it:

`R=(V)/(I)`

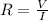
Replace V=120V and I=8.33A to find the resistance of the heater:

`R=(120V)/(8.33A)=14.4\Omega`

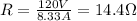
Therefore, the resistance of the heater is 14.4 Ohm.

Part F)

Assuming that the resistance of the heater does not change, we can isolate I from the definition of resistance and replace V=220V to find the current tha would pass through the heater if plugged into a 220V circuit:

`I=(V)/(R)=(220V)/(14.4\Omega)=15.3A`

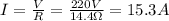
Since 15.3 A is more than 10A, then, the heater will burn out.