Solving the system of equations by using substitution, we have:
6r + 7s = –1 Equation(1)
2r + 4s = –12 Equation(2)
6r= -1 - 7s (Subtracting 7s from both sides of equation 1)
r= -1/6 - (7/6)s (Dividing by 6 on both sides of the equation 1)
Replacing r=-1/6 - (7/6)s in equation 2 we have:

Replacing s=-7 in the equation r= -1/6 - (7/6)s , we have:
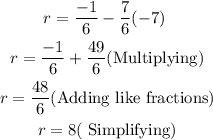
The solution of the sytem of equations is: r=8 and s=-7