Question

The angle of elevation from a ship to the lighthouse is 25 degrees. If the ship is 1500 meters from the lighthouse, how tall is the lighthouse? Answer to the nearest tenth of a meter. Draw a picture, write a equation, and solve.

Answer 1

We know that

• The angle of elevation is 25 degrees.

,

• The ship is 1500 meters from the lighthouse.

Let's make a diagram to visualize the problem.

Where x represents the height of the lighthouse. Let's use a trigonometric reason to find x.

In this case, it's convenient to use the tangent reason.

`\tan \theta=\frac{\text{opposite leg}}{adjacent\text{ leg}}`

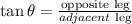
The opposite leg is x, the adjacent leg is 1500, and the angle is 25.

`\tan 25=(x)/(1500)`

To solve for x let's multiply each side by 1500.

`\begin{gathered} 1500\cdot\tan 25=1500\cdot(x)/(1500) \\ x\approx699.5m \end{gathered}`

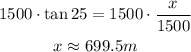
Therefore, the lighthouse is 699.5 meters tall.