Question

Physics - Standing wave modes on a string - assignment

Answer 1

a)

When a standing wave propagates through a string, the length of the string is a multiple of half the wavelength:

`L=n\cdot(\lambda_n)/(2)`

The factor n corresponds to the number of the harmonic. Then, the first harmonic is given by the condition n=1:

`\begin{gathered} L=(\lambda_1)/(2) \\ \Rightarrow\lambda_1=2L \end{gathered}`

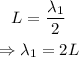
As we can see, the wavelength of the first harmonic is two times the length of the string.

Then, the wavelength of the first harmonic can be found by replacing the length L=63cm:

`\lambda_1=2*63cm=126cm`

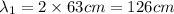
b)

The product of the wavelength and the frequency is the speed of the wave:

`v=\lambda f`

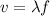
Replace λ=126cm=1.26m and f=330Hz to find the speed of the wave on the E-string:

`v=(1.26m)(330Hz)=415.8(m)/(s)\approx416(m)/(s)`

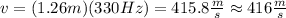
c)

The frequency of the n-th harmonic is given by:

`f_n=(v)/(\lambda_n)`

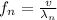
On the other hand:

`\lambda_n=(2L)/(n)`

Then:

`f_n=(v)/(2L)* n`

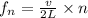
Notice that v/2L is the frequency of the first harmonic (fundamental frequency). Then:

`f_n=f_1* n`

Replace the fundamental frequency of 330Hz and n=2,3,4 to find the second, third and fourth harmonic frequencies:

`\begin{gathered} f_2=330Hz*2=660Hz \\ f_3=330Hz*3=990Hz \\ f_4=330Hz*4=1320Hz \end{gathered}`

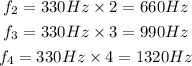
d)

Replace n=3 into the expression for the wavelength of the n-th harmonic to find the wavelength of the third harmonic:

`\lambda_n=(2L)/(n)=(2*63cm)/(3)=(126cm)/(3)=42cm`

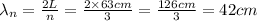
Therefore, the answers are:

a) 126cm

b) 416m/s

c) 660Hz, 990Hz, 1320Hz

d) 42cm