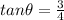Given:

Required:
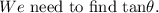
Step-by-step explanation:
Use the Pythagorean theorem to find the value of a.
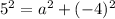
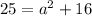
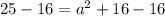
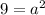
Take square root on both sides.
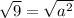
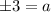
Here a is negative since the angle is in Quadrant III.
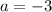
Consider the tangent.
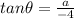
Substitute a =-3 in the equation.
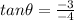
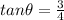
Final answer: