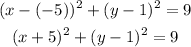Question

I need to know the answer and how to slove it step by step

Answer 1

`(x+5)^2+(y-1)^2=9`

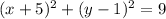
Step-by-step explanation

We want to find the equation that represents the circle given.

The general form for the equation of a circle is:

`(x-a)^2+(y-b)^2=r^2`

where (a, b) = center of the circle

r = radius of the circle.

From the graph, the center of the circle, K, is given as (-5, 1)

To find the radius of the circle, we have to find the distance from the center of the circle to any point on its circumference.

Let us use (-2, 1)

The formula for distance between two points is given as:

`D=\sqrt[]{(x_2-x_1)^2+(y_2-y_1)^2}`

Therefore, the radius is:

`\begin{gathered} r=\sqrt[]{(-2-(-5))^2+(1-1)^2}=\sqrt[]{(-2+5)^2+(1-1)^2} \\ r=\sqrt[]{3^2+0^2}=\sqrt[]{3^2} \end{gathered}`

From there, we can find the square of both sides of the equation to find r²:

`\begin{gathered} r^2=3^2 \\ r^2=9 \end{gathered}`

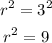
Therefore, the equation of the circle in the given graph is:

`\begin{gathered} (x-(-5))^2+(y-1)^2=9 \\ (x+5)^2+(y-1)^2=9 \end{gathered}`