We will solve this problem thus:
The Area of rectangle A is:
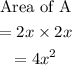
The Area of rectangle B is:
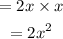
The area of the largest rectangle is:
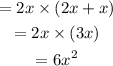
To find the fraction of rectangle A in relation to the largest rectangle, we will proceed thus:

So we can say that the area of rectangle A is 2/3 times the area of the largest rectangle.