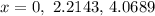Question

Use trigonometric identities, algebraic methods, and inverse trigonometric functions, as necessary, to solve the following trigonometric equation on the interval [0, 21).Round your answer to four decimal places, if necessary. If there is no solution, indicate "No Solution."--5cos(2x) + 4cos(x) + 1 = 0

Answer 1

Given:

`-5\cos (2x)+4\cos (x)+1=0`

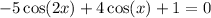
Let's solve the equation over the interval:

`\lbrack0,2\pi)`

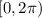
To find the equation over the interval, apply the double angle identity:

`\begin{gathered} -5(2\cos ^2(x)-1)+4\cos x+1=0 \\ \\ -10\cos ^2(x)+5+4\cos x+1=0 \end{gathered}`

Combine like terms:

`\begin{gathered} -10\cos ^2(x)+5+1+4\cos x=0 \\ \\ -10\cos ^2(x)+6+4\cos x=0 \end{gathered}`

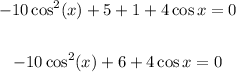
Factor:

`2(-5\cos (x)-3)(\cos (x)-1)=0`

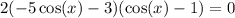
Set the individual factors to zero and solve:

`\begin{gathered} -5\cos (x)-3=0 \\ -5\cos (x)=3 \\ \cos (x)=-(3)/(5) \\ \\ \text{Take the cos inverse of both sides:} \\ x=\cos ^(-1)(-(3)/(5)) \\ \\ x=2.2143 \end{gathered}`

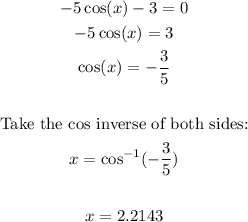
Also, the cosine function is negative in the second and third quadrants.

Subtract the reference angle from 2π to find the other angle:

`\begin{gathered} x=2\pi-2.2143 \\ x=4.0689 \end{gathered}`

Set the second factor to zero anmd solve for x:

`\begin{gathered} \cos x-1=0 \\ \cos x=1 \end{gathered}`

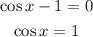
Take the inverse cosine of both sides:

`\begin{gathered} x=\cos ^(-1)(1) \\ \\ x=0 \end{gathered}`

The cosine function is positive in quandrant I and IV, to find the other angle, subtract the reference angle from 2π.

`\begin{gathered} x=2\pi-0 \\ x=2\pi \end{gathered}`

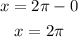
Let's find the period of the function:

`(2\pi)/(b)=(2\pi)/(1)=2\pi`

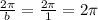
Since the period is 2π, values will repeat every 2π radians in all direction.

Here, we have the interval: [0, 2π).

This means 2π is not an included solution.

Therefore, the solutions to the given equation over the interval are:

`x=0,\text{ 2.2143, 4.0689}`

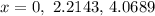
ANSWER:

`x=0,\text{ 2.2143, 4.0689}`