Question

A triangle has side lengths 6, 11 and 14. Is it acute, obtuse or right?xI✓Submit

Answer 1

We have a triangle with the following side lengths

6, 11, and 14

Let a = 6, b = 11, and c = 14

A triangle is said to be an "acute" triangle if the following relation holds true

`c^2Let us check if the above relation is true or not[tex]\begin{gathered} 14^2<6^2+11^2 \\ 196^{}<36^{}+121^{} \\ 196<157 \end{gathered}`

As you can see, the above relation is not true.

This is rather "obtuse" triangle

`c^2>a^2+b^2`

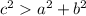
The above relation holds true in this case

`\begin{gathered} c^2>a^2+b^2 \\ 14^2>6^2+11^2 \\ 196>36+121 \\ 196>157 \end{gathered}`

So, this obtuse triangle will look like below

The angle opposite the longest side will be greater than 90°