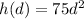Solution:
Since the height of the freefall is parabolic as shown below,
the standard form of expressing a parabola is given as
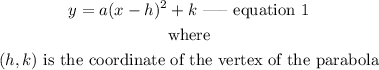
Step 1: Evaluate the coordinate of the vertex of the parabola.
In the above graph, the coordinate of the vertex is (0,0).
Thus,

Step 2: Substitute the values of h and k into equation 1.
Thus,
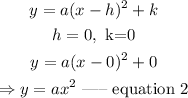
Step 3: Select a point on the curve, and substitute the values of x and y into equation 2, to obtain a.
Thus, using the point (0.2, -3) as shown above, where x=0.2 and y=-3, we have
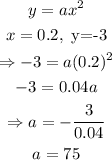
Substitute the value of a into equation 2.
Thus,
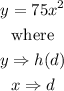
Hence, the function that describes these coordinates is expressed as