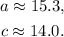To solve this question we will use the sine Law:
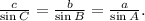
To find the measure of angle A, recall that the interior angles of a triangle add up to 180 degrees, therefore:
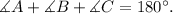
From the given data we know that:

Therefore:
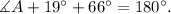
Solving the above equation for angle A we get:
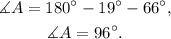
Now, from the given data we get that b=5, therefore:
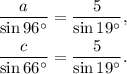
Solving the above equations for a and c respectively we get:
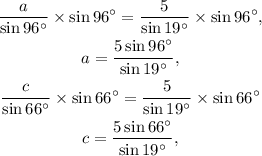
Therefore:
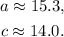
Answer: