Doug and Carol live 787.5 miles apart.
Distance = 787.5 miles
They start at the same time and travel toward each other.
Doug's speed is 7 mph greater than Carol's.
Let C represents Carol's speed then we can write
D = 7 + C
If they meet in 7.5 hours, find their speeds.
time = 7.5 hours
Recall that the relation between speed, time and distance is given by
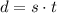
Let's plug the given values
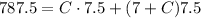
Where C is Carol's speed, (7 + C) is Doug's speed and 7.5 is the time in hours.
Simplify the equation
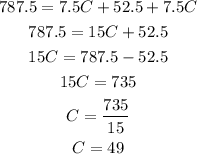
So that means Carol's speed is 49 mph
Doug's speed is
D = 7 + 49 = 56 mph
Doug's speed is 56 mph