Given:
mean/average = 100,000
Standard deviation = 10,000
raw data = 120,000
Find: the percentage of salespeople expected make an annual sale of 120,000
Solution:
To be able to get the percentage, we need to convert the raw value to z-score first. The formula is:
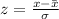
where x = raw data, bar x = mean, and σ = standard deviation.
Since we have those values in the given data, let's plug it in to the formula.
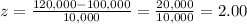
Hence, the z-score of the given raw data is 2.00.
Based on the standard normal distribution table, the area covered by z = 2.00 or greater is 0.0228.
Therefore, the percentage of the salespeople who are expected to make an annual sales of 120,000 is only 2.28%.