Question

In the diagram below, AD bisects ZCAB, m_ADB = 100° andmZB = 48°. Find mZCAD.сD100°A48B

Answer 1

Line AD bisecting ΔCAB results in two triangles. The first triangle ΔADB has angle measurements m_ADB = 100° and

m B = 48°. The total angle of a triangle is 180 degrees. Hence, the measurement of angle A on the triangle ΔADB is

`\begin{gathered} \angle A+\angle B+\angle ADB=180 \\ \angle A=180-\angle B-\angle ADB \\ \angle A=180-100-48=32 \end{gathered}`

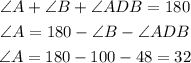
The triangle ΔCAD has no given angles on it. But we can find first the angle of m CDA by knowing that angle CDA and angle ADB as supplementary angles.

`\begin{gathered} \angle CDA+\angle ADB=180 \\ \angle CDA=180-\angle ADB \\ \angle CDA=180-100=80 \end{gathered}`

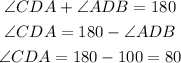
Using sine law, the angles DAB, CAD, BDA, and CDA are related as

`(\angle DAB)/(\angle BDA)=(\angle CAD)/(\angle CDA)`

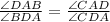
Solve for the value of angle CAD

`\angle CAD=(32)/(100)*80=25.6`

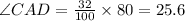
Hence, angle CAD has an angle measurement of 25.6°.