In order to calculate the length of the third side, let's use the law of cosines:
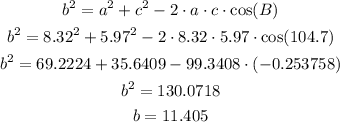
Now, let's calculate angle A using law of sines:
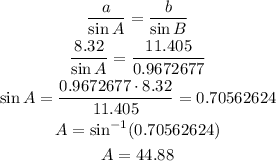
Since the sum of internal angles in any triangle is equal to 180°, we have:
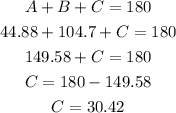
Therefore the answer is option A:
A = 44.9°, C = 30.4°, b = 11.41 m