SOLUTION
Given the question in the image, the following are the solution steps to answer the question.
STEP 1: Place the numbers from the least to greatest
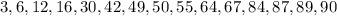
STEP 2: Find the median
The median is the number in the middle position. The middle position will be the 8th and the number there is 50.
Median is 50
STEP 3: Find the lower quartile
The lower quartile is the median of the data set to the left of the median

16 is the lower quartile
STEP 4: Find the upper quartile
The upper quartile is the median of the data set to the right of the median
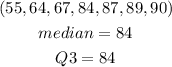
84 is the upper quartile
STEP 5: Find the maximum and minimum of the data
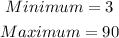
STEP 6: Find the Interquartile range
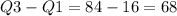
Hence, the 5-number summary is given as:
Minimum = 3
Lower quartile = 16
Median = 50
Upper quartile = 84
Maximum = 90