Question

A man runs at a speed of 4 m/s to overtake a standing bus. When he is om behind the door (t=0), the busmoves forward and continues with a constant acceleration of 1.2 m/s^2. How long does it take for the man to gain the door?

Answer 1

In order to determine the time the man takes to reache the door, consider that the motion of the man is with constant speed and the motion of the bus is accelerated.

Use the following formulas for the distance traveled by both man and bus in the time t:

`\begin{gathered} x=vt \\ x^(\prime)=x_0+v_ot+(1)/(2)at^2 \end{gathered}`

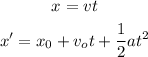
where:

v: man speed = 4 m/s

t: time that man takes to reach the bus = ?

xo: initial position = 0m

a: bus acceleration = 1.2 m/s^2

vo = initial speed of the bus = 0 m/s

the man reaches the door of the bus when x'=x, that is, when both man and bus traveled the same distance. Then, you have:

`\begin{gathered} x=x^(\prime) \\ vt=(1)/(2)at^2 \end{gathered}`

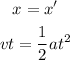
solve the previous equation for and replace the values of the parameters:

`t=(2v)/(a)=(2((4m)/(s)))/((1.2m)/(s^3))=(20)/(3)s\approx6.66s`

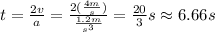
Hence, the man takes approximately 6.66s to reach the door of the bus