The given system of inequalities is

At first, we will draw each line using 2 points on it
Remember:
1. If the sign of inequality has equal, then the line must be solid
2. If the sign of inequality has no equal sign, then the line is dashed
3. If the sign of inequality is >, then the shaded area is above the line
4. If the sign of inequality is <, then the shaded area is under the line
Now, let us find the points on the lines

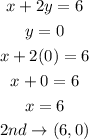
We will do the same with the second line
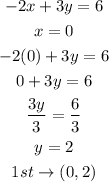
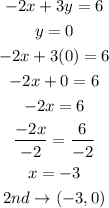
We will draw the graph using the points above
The red graph represents inequality (1)
The red line is dashed
The shaded area is above the line
The blue graph represents the inequality (2)
The blue line is solid
The shaded area is below the line
The area of the solution is the area that contains the two colors together
The area of the solution is marked by a star.